
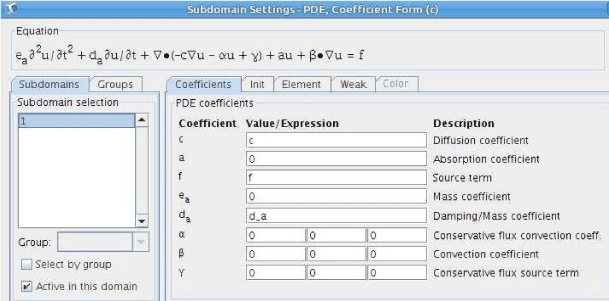
#Where can i find discretization section in comsol 5.3 full
Therefore, it is the purpose of this work to develop a full non-linear heat transfer model, and then use it to try and infer the parameters of a sample of known composition, through uncertainty quantification, thus opening the possibility for using such model for inference of temperature of a sample of unknown composition. It could be therefore useful to use a non-linear model, using a numerical approach, for which one continues to develop the mathematical model. Still other applications for active thermography use the full heat transfer equation, but no uncertainty quantification is presented ( like in (Cannas, et al.,).
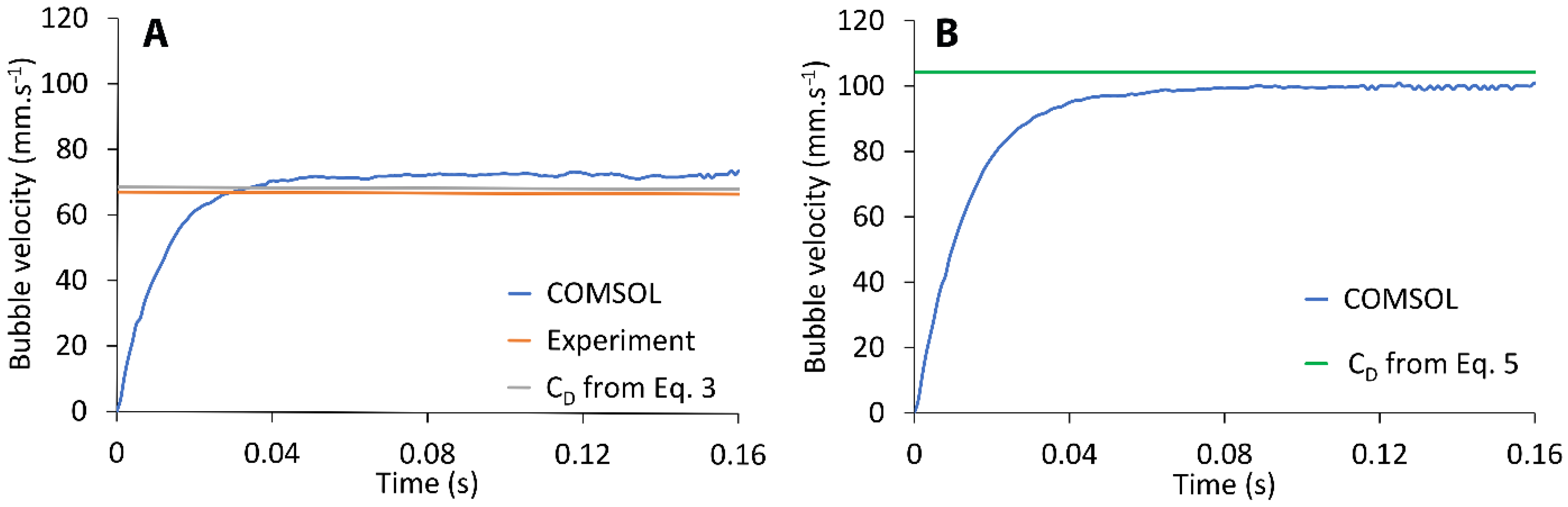
Other works (like in (Benzerrouk)), describe, but not use the full heat transfer equation. Successful as it is the approximation mentioned in (and other similar to this, like in, the usage of logarithms in one coordinate implies that some information is lost, as irregularities are flatten down. C p for thin slabs of known thickness, because it is obtained from τ by least squares fit of experimental curves of θ versus t Eq.3, and normally semi-log plots are used to avoid uncertainties due to deviations from the theoretical model.Eq.3 has been used to calculate the specific heat capacity Τ = t h ⋅ ρ m ⋅ C p 2 h (4) the relaxation (transient) time of the system. Θ ↑ = Φ A h ( 1 − exp ( − t τ ) ) (3) A is the total area of the sample and ∂ θ ∂ t + h ρ m ⋅ C p ⋅ t h θ − Φ ρ m ⋅ C p ⋅ V = 0 (2) is a linear ordinary differential equation with constant coefficients, where h is the total heat transfer coefficient (a linearized sum coming from convection, conduction and radiation), ρm is the material density, C p is the specific heat capacity, and V is the volume of the sample the particular units used in this work will be mentioned later on. If we want to calculate the rise of temperature, θ, of the surfaces that are not in contact with an incident laser, we must express the heat term of Eq.1 as a function of that increase, which can be expressed as
∂ Q ∂ t = Φ − q (1) q is a term specified by the sum of the power losses by radiation, convection and conduction.
The variation with time, t, of the heat generated in the sample, Q, due to the absorption of light of incident power Φ, is given by On the opposite side, its temperature can be monitored as a function of time, for example with a thermocouple. Problem possing and objectivesĬonsider that a thin slab of a solid sample of thickness th is heated with a light beam (laser) that is uniformly focused onto one or more of its surfaces (boundaries). This is the case of thermography for cancer research, aiming at substituting the painful mammograms with much less invading techniques. Also, there are instances where the sample cannot or must not be destroyed. The spatial thermal parameters of a sample may differ when this has a concrete shape and dimensions, as opposite of measuring fine grinding of the material this sample is made of. The set of performed observations (measurements), may be thus modeled through a heath-transfer, partial differential equation (transport equation). Thus, it is used thermal data from a heated sample with a laser beam, on its original state, that is, without reducing it to dust or any other destructive process. Given the possibility of controlling the intensity of the external energy source, the artificial thermal excitation can reach deeper atoms in the object, and therefore information can be obtained from more internal layers. In active thermography, an external energy source is required for artificial thermal excitation. The inverse problem is that given experimental results, determine the possible value of a coefficient of the differential equation that models the phenomenon. The need of a mathematical model, particularly one that is suitable for an inverse problem, is the central matter of this project. From the parameters of those heat mechanisms, it is possible to infer the heat properties of the sample. Active thermography uses a heat source in order to induce absorption of such heat into a sample of a possibly unknown composition, which then will dissipate through several heat transfer mechanisms.


 0 kommentar(er)
0 kommentar(er)
